Methods

The focus of this chapter is to understand the dependence of the global topography (not just the low energy regions as in Chapter 2) of the PES on the range of the potential. To achieve this aim we again use the Morse potential; the analysis of the contributions to the potential energy given in §2.2.1 will be extremely important for understanding the range dependence of the thermodynamics.

  

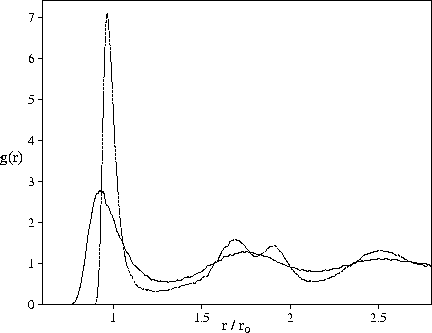
Figure 4.2: Bulk liquid radial distribution functions from a set of instantaneous co-ordinates (solid line) and the resulting minima after quenching (dashed line). The system is a periodically repeated cubic cell containing 256 atoms interacting via a Morse potential with $\rho_0$=6.

In the present examination of the PES we will generally focus upon minima. As we noted in Chapter 3 this `inherent structure' approach[148] allows one to analyse the thermodynamics in detail by separating the effects of the energetic distribution of local minima from the effects of thermal motion within the minima. Our analysis of the thermodynamics here will be more qualitative, simply concentrating on the distribution of minima. By removing the effects of vibrational noise this approach also allows a much clearer picture of liquid structure. This point is illustrated in Figure 4.2 by a comparison of the radial distribution functions derived from a set of instantaneous configurations of a bulk Morse liquid and the configurations of the corresponding minima. The peaks in the radial distribution function for the minima are much sharper, showing that the structure is much more well-defined. Of particular interest is the split second peak for the minima radial distribution function. This feature only develops in the instantaneous radial distribution function for supercooled liquids and glasses[187]. It is not too surprising that minimization and reducing the temperature have similar effects, and this helps to emphasize the structural connection between the liquid and the glass.