Introduction

In a recent paper Hagen et al. posed the question, `Does C₆₀ have a liquid phase?'[177] A liquid-vapour transition can only occur between the triple point temperature, below which only the solid and vapour are stable, and the critical temperature, above which there is only one fluid phase (Figure 4.1a). So, if the critical temperature is lower than the solid-fluid coexistence temperature at the critical density (Figure 4.1b), the liquid phase is thermodynamically unstable[178]. The answer to Hagen's question, though, has not yet been unequivocally answered: theoretical calculations of the phase diagram predict the liquid phase of bulk C₆₀ is either unstable[177] or only marginally stable[179,180] depending on the simulation technique used, whilst experiment seems to suggest that C₆₀ molecules are thermally unstable at the relevant temperatures[181].

  

Figure 4.1: Temperature/density phase diagrams when (a) there is a stable liquid phase, and (b) the liquid phase is thermodynamically unstable. S, L, V and F stand for solid, liquid, vapour and fluid, respectively. T_c is the critical temperature, T_t is the triple point temperature, T_sc is the solid-fluid coexistence temperature at the critical density, $\rho_c$ is the critical density, and $\rho_{fc}$ is the density of the fluid which coexists with the solid at the critical temperature. The dashed lines are in (a) the metastable solid-fluid coexistence line and in (b) the metastable liquid-vapour coexistence line. For (a) T_c>T_t>T_sc and $\rho_c<\rho_{fc}$ and for (b) T_c<T_sc and $\rho_c\gt\rho_{fc}$. The latter are the necessary and sufficient conditions for there to be no stable liquid phase. The dotted lines are to guide the eye.

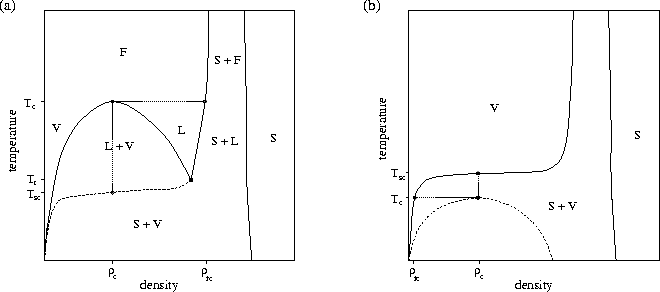
In contrast to C₆₀, for which the intermolecular potential is very short-ranged with respect to the equilibrium pair separation, the critical temperature for sodium is about seven times larger than the triple point temperature because of the long-ranged interatomic forces. The results for C₆₀ have led to a flurry of studies examining the effect of the range of the potential on the phase diagram[182,183,184,185]. These investigations have clearly shown that as the range of attraction decreases, the difference between the triple and critical temperatures decreases until the critical temperature drops below the triple point and the liquid phase disappears. Similar effects have previously been noted for mixtures of spherical colloidal particles and non-adsorbing polymer by theory[120], simulation[186] and experiment[121,122,123]. For such systems, the size of the polymer can be used to vary systematically the range of attraction between the colloidal particles.

Although the phenomenology of the range-dependence of the liquid phase stability is clear, a structural explanation has not been given. In this chapter we provide such a microscopic view by relating the above effects to fundamental changes in the topography of the PES (§4.3) and by making a detailed connection between these changes and liquid structure (§4.4). By studying both clusters and bulk we can address questions concerning the emergence of the phase-like forms of clusters and their evolution to the bulk limit. Detailed simulations of the thermodynamic properties of a 55-atom cluster (§4.5) confirm that our results can explain the range-dependence of the thermodynamics. We can also suggest an explanation for the transition from electronic to geometric magic numbers observed in the mass spectra of sodium clusters[53] (§4.6), showing that the simple approach we describe here can provide insight into a diverse set of phenomena.