


Next: Decahedral clusters
Up: Results
Previous: Results
Home: Return to my homepage
The growth sequence that leads from the 7-atom pentagonal bipyramid to the 13-atom icosahedron
is shown in Figure 2.8.
The growth occurs by the capping of the faces on one half of the bipyramid.
For 11 and 12, related structures become the global minimum at short range.
Firstly, a symmetry breaking of structures 11C and 12B occurs.
This results in the loss of one (11D) or two (12C) nearest-neighbour bonds,
but also allows the other nearest-neighbour contacts to relax closer to the
pair equilibrium distance.
At still larger  , structures 11E and 12D, which involve capping of the faces on both sides of the
pentagonal bipyramid, become lowest in energy.
These structures have fewer nearest neighbours, but they are also less strained.
All the structures shown in Figure 2.8 are polytetrahedral,
in the sense that the whole of the cluster can be divided into tetrahedra with atoms at
the vertices.
, structures 11E and 12D, which involve capping of the faces on both sides of the
pentagonal bipyramid, become lowest in energy.
These structures have fewer nearest neighbours, but they are also less strained.
All the structures shown in Figure 2.8 are polytetrahedral,
in the sense that the whole of the cluster can be divided into tetrahedra with atoms at
the vertices.
Figure 2.9:
Atomic positions for the two possible overlayers of the icosahedron, anti-Mackay (left) and Mackay (right).
These are shown for a single face of the icosahedron.
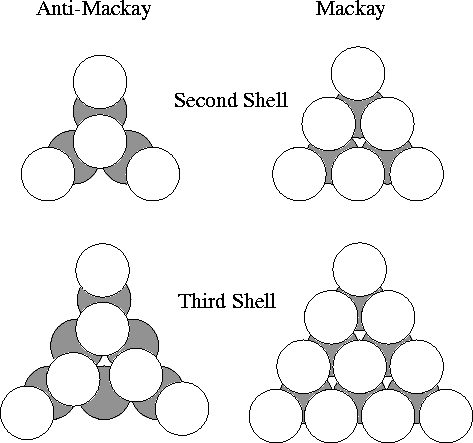 |
Addition of atoms to the 13-atom icosahedron can occur in two ways.
The two types of overlayer that result are illustrated in Figure 2.9.
One growth mode continues the fcc packing of the twenty strained tetrahedra making up the icosahedron,
and leads to the 55-atom Mackay icosahedron (Figure 2.1b).
This growth mode introduces octahedral interstices, and so the resulting structures
are no longer polytetrahedral.
The other growth mode involves sites which are hcp with
respect to the tetrahedra, and has been termed the anti-Mackay overlayer.
For growth on the 13-atom icosahedron, this overlayer preserves polytetrahedral character.
Each of the vertices of the original icosahedron becomes icosahedrally coordinated,
and the structure that results from the completion of this overlayer, 45A, is a
rhombic tricontahedron; it is an icosahedron of interpenetrating icosahedra.
Interestingly, the rhombic tricontahedron is the face-dual of the
truncated icosahedron made famous by C60; indeed, it is even a particularly stable shell for the
coverage of a C60 molecule by alkaline earth metal atoms[96].
In previous studies, the anti-Mackay overlayer has been referred to as
the polyicosahedral[43,44,45]
or the face-capping overlayer[50].
Such names are reasonable for growth on the 13-atom icosahedron, but are confusing for growth
on larger Mackay icosahedra.
The icosahedral structures with an anti-Mackay overlayer are illustrated in Figure 2.10
and those with an Mackay overlayer in Figure 2.11.
Growth from the 13-atom icosahedron begins in the anti-Mackay
positions, because these do not include any low-coordinate edge sites, thus
giving the structures a larger nnn.
However, at some critical size the Mackay overlayer becomes lower in energy,
because of the larger strain energies associated with the anti-Mackay overlayer.
Further growth then leads to the next Mackay icosahedron.
The size at which this change occurs depends on the range of the potential;
it is given by the dashed line of negative slope that divides the
icosahedral region of the phase diagram (Figure 2.5).
The crossover size increases with the range of the potential;
a long-ranged potential can accommodate the greater strain associated with the
anti-Mackay overlayer more easily.
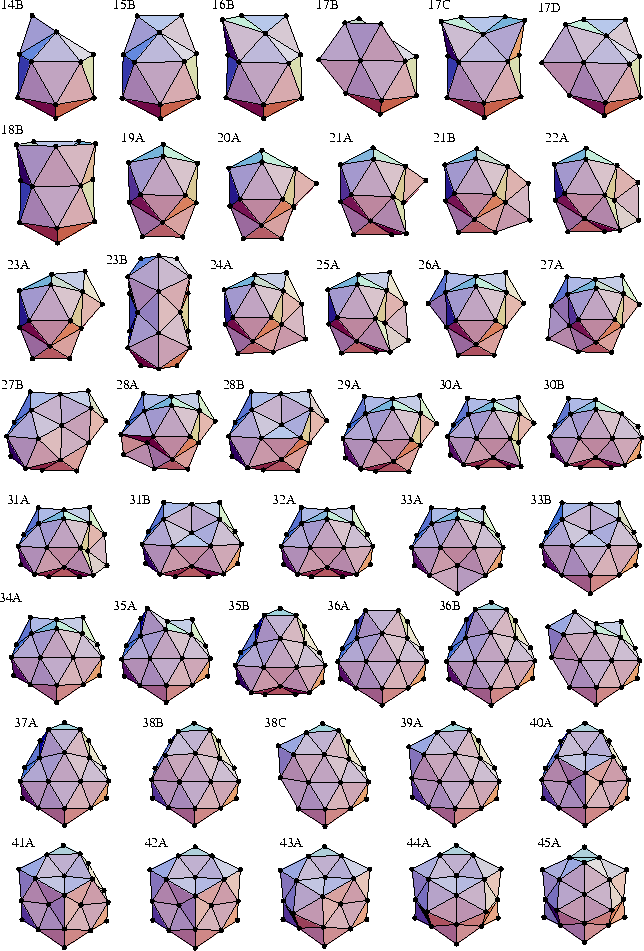
Figure 2.10:
Icosahedral global minima formed by growth of an anti-Mackay overlayer on the 13-atom icosahedron,
except structure 23B which is composed of two face-sharing icosahedra.
 |
At 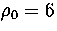 the Mackay overlayer is occupied for
the Mackay overlayer is occupied for 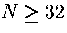 .
This compares to
.
This compares to 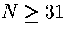 for LJ clusters; as expected the results are very similar.
In ab initio molecular dynamics calculations for lithium clusters[97],
the anti-Mackay overlayer is lowest in energy up to N=45.
Similarly, polytetrahedral structures were observed in a tight-binding study of sodium clusters
up to the largest size considered N=34 (for which 34A was the most stable structure)[98].
These effects are nicely explained by the long range of alkali metal potentials.
for LJ clusters; as expected the results are very similar.
In ab initio molecular dynamics calculations for lithium clusters[97],
the anti-Mackay overlayer is lowest in energy up to N=45.
Similarly, polytetrahedral structures were observed in a tight-binding study of sodium clusters
up to the largest size considered N=34 (for which 34A was the most stable structure)[98].
These effects are nicely explained by the long range of alkali metal potentials.
Especially stable structures with an anti-Mackay overlayer occur when the icosahedral
coordination of a vertex atom is complete.
These structures give rise to the peaks in 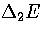 at N=19, 23 26, 29, 34 and 45 for
at N=19, 23 26, 29, 34 and 45 for  =3;
only the first four of these peaks are seen at
=3;
only the first four of these peaks are seen at  =6, and only the first at
=6, and only the first at  =10 (Figure 2.6).
Some of these magic numbers have been observed in mass spectroscopic
studies of rare gases[47,48] and even barium[99].
The centres of the icosahedra in these structure form a dimer for 19A, an equilateral triangle for 23A,
a tetrahedron for 26A, a trigonal bipyramid for 29A, a pentagonal bipyramid for 34A, and an icosahedron for 45A.
At a number of sizes there is more than one global minimum with an anti-Mackay overlayer.
The transitions between these structures are related to slight differences in the value of
=10 (Figure 2.6).
Some of these magic numbers have been observed in mass spectroscopic
studies of rare gases[47,48] and even barium[99].
The centres of the icosahedra in these structure form a dimer for 19A, an equilateral triangle for 23A,
a tetrahedron for 26A, a trigonal bipyramid for 29A, a pentagonal bipyramid for 34A, and an icosahedron for 45A.
At a number of sizes there is more than one global minimum with an anti-Mackay overlayer.
The transitions between these structures are related to slight differences in the value of
 .Also illustrated in Figure 2.10 is structure 23B, which is made up of two face-sharing
icosahedra.
It can be formed from structure 17B by the addition of six atoms not to the icosahedron but to the atoms
which are part of the overlayer.
.Also illustrated in Figure 2.10 is structure 23B, which is made up of two face-sharing
icosahedra.
It can be formed from structure 17B by the addition of six atoms not to the icosahedron but to the atoms
which are part of the overlayer.
Figure 2.11:
Icosahedral global minima formed by growth of an Mackay overlayer on the 13-atom icosahedron.
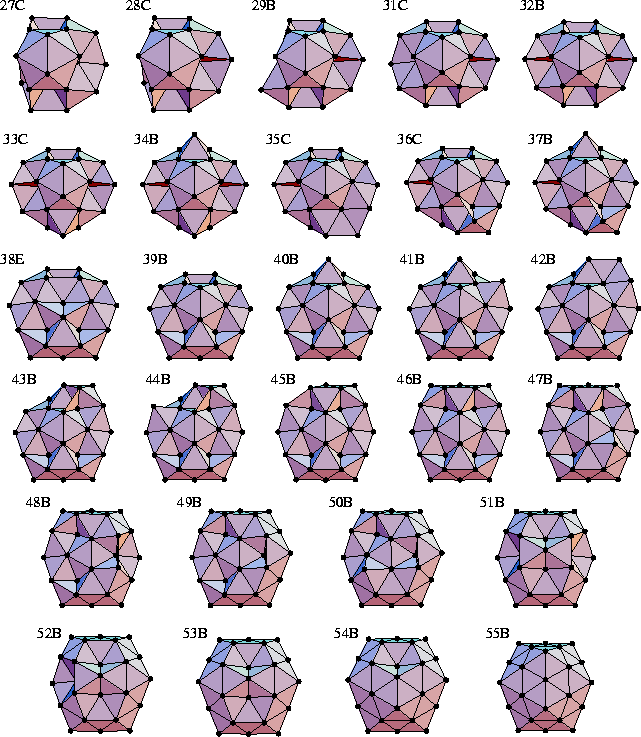 |
The first global minimum with a Mackay overlayer occurs at N=27.
Especially stable structures occur at those sizes for which complete faces of the 55-atom Mackay
icosahedra are missing. These structures give rise to the peaks at N=39, 46 and 49 for  =6 and
correspond to 5, 2 and 1 missing faces, respectively. Again, these magic numbers have
been seen in mass spectra of rare gases[48].
=6 and
correspond to 5, 2 and 1 missing faces, respectively. Again, these magic numbers have
been seen in mass spectra of rare gases[48].
Structure 38E has an atom missing from a vertex of the
original 13-atom icosahedron to allow the overlayer to complete a particularly stable form.
This structure was not found in Northby's study of icosahedral LJ clusters,
even though it is the lowest energy LJ icosahedral cluster[73].
Figure 2.12:
Icosahedral global minima formed by growth from the 55-atom Mackay icosahedron.
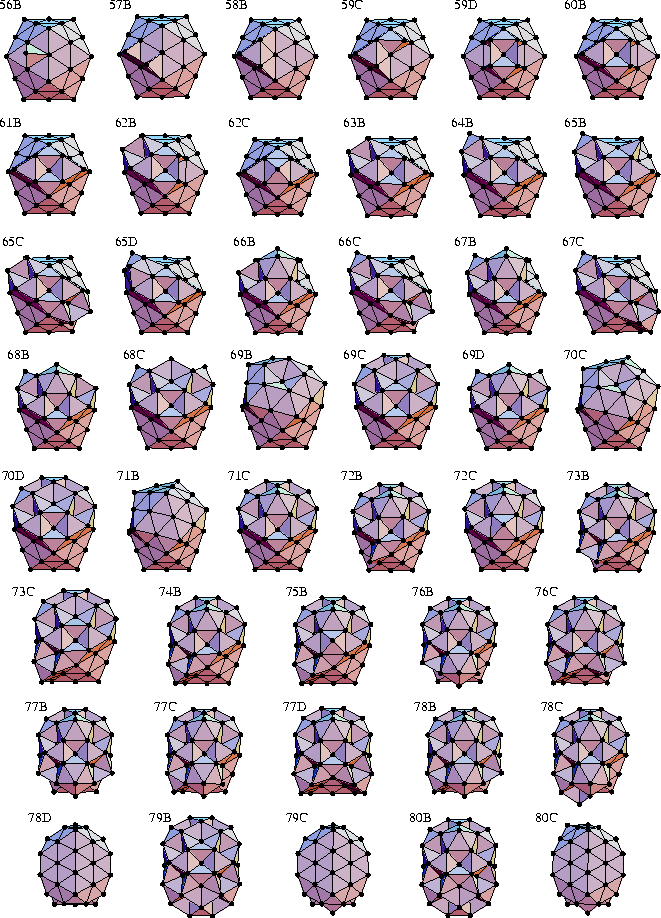 |
The icosahedral global minima with more than 55 atoms are shown in Figure 2.12.
As for the 13-atom icosahedron, growth initially occurs at the anti-Mackay sites, because this
results in structures with larger nnn.
Completion of this overlayer occurs for a cluster with 127 atoms.
The vertex atoms of the 55-atom Mackay icosahedron become icosahedrally coordinated, but
the edge atoms have a decahedral (13B) coordination shell (this is clearly visible for 59C)
leading to the half octahedra that are visible in the surface layers of the anti-Mackay clusters.
The most prominent peak in 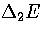 due to an anti-Mackay structure occurs at N=71 for
due to an anti-Mackay structure occurs at N=71 for  =6.
This corresponds to an overlayer which completely covers the five faces surrounding a vertex
of the underlying icosahedron. There are smaller peaks at N=58, 61, and 64, which
correspond to complete coverage of one, two and three faces, respectively.
=6.
This corresponds to an overlayer which completely covers the five faces surrounding a vertex
of the underlying icosahedron. There are smaller peaks at N=58, 61, and 64, which
correspond to complete coverage of one, two and three faces, respectively.
Similar to 38E, structure 69C has a vertex atom missing from the underlying Mackay icosahedra.
There are also structures (62C, 65D, 72C and 75B) where an atom is added to the surface of the overlayer
rather than to the Mackay icosahedra.
The first structure in this size range with a Mackay overlayer is 78D.
It is expected that the size at which the crossover from an anti-Mackay to a Mackay overlayer
again increases with the range of the potential, but to confirm this prediction the study would need
to be extended to larger sizes.
There are also three icosahedral global minimum, 69B, 70C and 71B, which do not fit neatly into
either the Mackay or anti-Mackay category.
The surface layer has a Mackay-like character, but it is not in correct
alignment with the underlying icosahedron.
The overlayer has been given a twist about one of the fivefold axis in order to
collapse some of the half octahedra at the edge of the
overlayer into trigonal bipyramids in a multiple diamond-square-diamond (DSD) process[100].
In fact for 71 at  =5, the lowest energy Mackay structure is a transition state
which mediates a multiple DSD rearrangement between two permutational isomers of 71B.
=5, the lowest energy Mackay structure is a transition state
which mediates a multiple DSD rearrangement between two permutational isomers of 71B.
Figure 2.7 provides an illuminating illustration of the variation of the icosahedral
energies with size.
The complete Mackay icosahedra represent narrow minima, which are separated by broad maxima
corresponding to structures with an incomplete outer shell.
At  =6 it is only near the top of these maxima that fcc and decahedral structures
begin to have similar energies, such as at N=38 and 75.
=6 it is only near the top of these maxima that fcc and decahedral structures
begin to have similar energies, such as at N=38 and 75.



Next: Decahedral clusters
Up: Results
Previous: Results
Home: Return to my homepage
Jon Doye
8/27/1997



![]() at N=19, 23 26, 29, 34 and 45 for
at N=19, 23 26, 29, 34 and 45 for ![]() =3;
only the first four of these peaks are seen at
=3;
only the first four of these peaks are seen at ![]() =6, and only the first at
=6, and only the first at ![]() =10 (Figure 2.6).
Some of these magic numbers have been observed in mass spectroscopic
studies of rare gases[47,48] and even barium[99].
The centres of the icosahedra in these structure form a dimer for 19A, an equilateral triangle for 23A,
a tetrahedron for 26A, a trigonal bipyramid for 29A, a pentagonal bipyramid for 34A, and an icosahedron for 45A.
At a number of sizes there is more than one global minimum with an anti-Mackay overlayer.
The transitions between these structures are related to slight differences in the value of
=10 (Figure 2.6).
Some of these magic numbers have been observed in mass spectroscopic
studies of rare gases[47,48] and even barium[99].
The centres of the icosahedra in these structure form a dimer for 19A, an equilateral triangle for 23A,
a tetrahedron for 26A, a trigonal bipyramid for 29A, a pentagonal bipyramid for 34A, and an icosahedron for 45A.
At a number of sizes there is more than one global minimum with an anti-Mackay overlayer.
The transitions between these structures are related to slight differences in the value of
![]() .Also illustrated in Figure 2.10 is structure 23B, which is made up of two face-sharing
icosahedra.
It can be formed from structure 17B by the addition of six atoms not to the icosahedron but to the atoms
which are part of the overlayer.
.Also illustrated in Figure 2.10 is structure 23B, which is made up of two face-sharing
icosahedra.
It can be formed from structure 17B by the addition of six atoms not to the icosahedron but to the atoms
which are part of the overlayer.

![]() =5, the lowest energy Mackay structure is a transition state
which mediates a multiple DSD rearrangement between two permutational isomers of 71B.
=5, the lowest energy Mackay structure is a transition state
which mediates a multiple DSD rearrangement between two permutational isomers of 71B.
![]() =6 it is only near the top of these maxima that fcc and decahedral structures
begin to have similar energies, such as at N=38 and 75.
=6 it is only near the top of these maxima that fcc and decahedral structures
begin to have similar energies, such as at N=38 and 75.