Structural information is of fundamental importance in addressing the chemical and physical properties of any system. Unfortunately, there is no direct experimental method for determining the structure of free clusters in molecular beams. Instead, one measures properties which depend on structure and employs models of the predicted favoured geometries. This approach has been combined with techniques such as electron diffraction,[1] mass spectral abundances,[2] chemical reactivity,[3] magnetism[4] and x-ray spectroscopy.[5] The inversion of the experimental data to obtain structural information, though, can be problematic, and always relies on comparisons with the predictions of structural models.
Sizes exhibiting special stability are known for certain morphologies and classes of interatomic potential.[6,7] The size-dependence of properties sometimes reveals these magic numbers, and thus enables a confident structural assignment to be made. However, often rather little is known about the structure between these magic numbers. One of the most powerful experimental techniques that addresses this deficiency is the flow-reactor approach which probes the chemical reactivity of size-selected clusters. For example, this method has been applied to nickel clusters to give detailed information for all sizes up to N=71.[8,9,10,11] The results show that around N=13 and N=55 the clusters are icosahedral, in agreement with the observed magic numbers in other experiments.[4,12,13] However, in the size range 29<N<48 only one structural assignment has so far been made because of the large number of possible geometries to be considered and the presence of multiple isomers.[11]
The theoretician can aid in the task of structural assignment by providing realistic candidate structures. Indeed, many studies have attempted to model specific clusters, but ab initio calculations are only feasible for small sizes, especially for transition metals, and so empirical potentials are often used. However, as is clear from the diversity of theoretical results obtained for nickel clusters,[14,15,16,17,18,19,20] consensus between methods is lacking and it is hard to know which (if any) of the results should be believed. Even with the simplified description of the interatomic interactions provided by an empirical potential, it can be an extremely difficult task to search the potential energy surface (PES) extensively enough to be confident that the global minimum has been found. Also, many empirical potentials are too complicated to provide an understanding of the relationship between the potential and the observed structure and so little physical insight is gained.
Therefore, to understand cluster structure there is a need for a hierarchy of theoretical models from the general to the specific. In the present study we use a simple model to understand the structural effects of the range of the potential, and so provide one part of the framework for understanding the physical basis of cluster structure. We are confident that we have found most of the global minima giving the most comprehensive model of cluster structure in the small size regime.
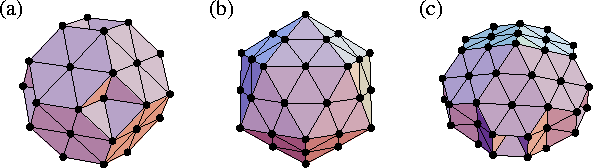
One of the most interesting aspects of cluster structure is the manifestation of non-crystallographic symmetries which arise from the absence of translational periodicity. In particular, many clusters are found to have fivefold axes of symmetry, including two of the three main types of ordered structure adopted by simple atomic clusters. Decahedra have a single fivefold axis of symmetry and are based on pentagonal bipyramids, while icosahedra have six fivefold axes of symmetry. The third morphology consists of close-packed clusters. Particularly stable examples of each type are illustrated in Fig. 1.
 |
All the above morpholgies have been observed experimentally. Many gas phase clusters have been shown to be icosahedral through the presence of the magic numbers associated with the Mackay icosahedra[21] in mass spectra: rare gases[22,23,24], metals,[13,25,26,27] and molecular clusters.[28,29] Icosahedral and decahedral structures are also commonly reported for metal clusters supported on surfaces,[30] and more recently fcc and decahedral clusters have been observed for gold clusters passivated by alkylthiolates.[31,32,33,34,35]
All three structural types are also exhibited by Lennard-Jones (LJ) clusters.
For N<1600 icosahedra are most stable; from this size up to 
decahedra are
most stable and above this fcc clusters.[36]
However, these changes do not occur abruptly.
The global minimum of 
is the fcc truncated octahedron[37,38] (Fig. 1(a))
and for at least six sizes with N<110 the global minimum is based upon a Marks
decahedron[7,38] (Fig. 1(c)).
In this paper we consider a potential with variable range to provide a model system
which exhibits a much greater diversity of structural behaviour than
LJ clusters in the small size regime.
Consequently, the results are relevant to a much wider range of systems.
There have been a number of previous studies on the effect of
the range of the potential on the structure and phase behaviour of small
clusters.[38,39,40,41,42,43,44,45]
These have shown that the number of minima and saddle points
on the PES increases as the range decreases--the PES becomes more
rugged[39,42,46,47]--and strained structures are destabilized.
The latter effect results in range-induced transitions between the ordered morphologies,[38]
and the destabilization and disappearance of the liquid phase as the range is
decreased.[41,42,48]
In a previous paper we examined Morse clusters containing up to
25 atoms and a selection of larger sizes.[38]
Here we consider Morse clusters in the size range 
atoms.
Some of the lowest energy structures given here supersede the results of the previous paper.