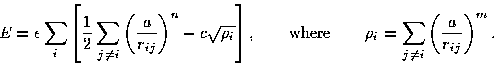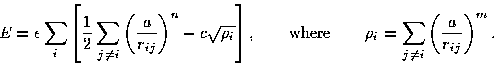The Sutton-Chen Potential
The Sutton-Chen (SC) potential has the form:
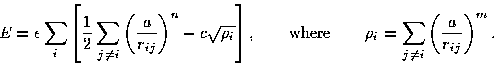
c is a dimensionless parameter,
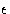 is a parameter with dimensions of energy, a is the lattice constant,
and m and n are positive integers with n>m.
We use the n, m and c parameters given by Sutton and Chen
for the metals Ag, Ni and Au; Rh has the same scaled parameters as Ag,
Cu the same as Ni and
Pt is the same as Au, so the corresponding results for
these metals can simply be obtained from their partners by rescaling.
For Ag and Rh n = 12, m = 6 and c=144.41,
for Ni and Cu n = 9, m = 6 and c=39.432,
for Au and Pt n = 10, m = 8 and c=34.408.
In the present calculations we employed reduced units with
is a parameter with dimensions of energy, a is the lattice constant,
and m and n are positive integers with n>m.
We use the n, m and c parameters given by Sutton and Chen
for the metals Ag, Ni and Au; Rh has the same scaled parameters as Ag,
Cu the same as Ni and
Pt is the same as Au, so the corresponding results for
these metals can simply be obtained from their partners by rescaling.
For Ag and Rh n = 12, m = 6 and c=144.41,
for Ni and Cu n = 9, m = 6 and c=39.432,
for Au and Pt n = 10, m = 8 and c=34.408.
In the present calculations we employed reduced units with
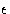 =1
and a=1.
The tabulated results may therefore easily be rescaled for any of
the above elements.
The appropriate energy is given simply by multiplying the reduced energy
by
=1
and a=1.
The tabulated results may therefore easily be rescaled for any of
the above elements.
The appropriate energy is given simply by multiplying the reduced energy
by 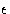 whilst the coordinates need to be multiplied by
a, i.e. the lattice constant.
The Sutton-Chen potential provides a reasonable description of
various bulk properties,
with an approximate many-body representation of the delocalized metallic bonding.
However, it does not include any directional terms, which are likely
to be important for transition metals with partially occupied d shells.
whilst the coordinates need to be multiplied by
a, i.e. the lattice constant.
The Sutton-Chen potential provides a reasonable description of
various bulk properties,
with an approximate many-body representation of the delocalized metallic bonding.
However, it does not include any directional terms, which are likely
to be important for transition metals with partially occupied d shells.
Reference: A. P. Sutton and J. Chen, Phil. Mag. Lett. 61, 139 (1990)
Jon Doye
11/5/1997